B.8 Contraction Problem
The contraction problem may be described by considering a general contracted ERI of  -type functions derived from the STO-3G basis set. Each basis function has degree of contraction
-type functions derived from the STO-3G basis set. Each basis function has degree of contraction  = 3. Thus, the ERI may be written
= 3. Thus, the ERI may be written
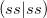 |
 |
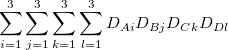 |
|||
 |
 |
![$\displaystyle \times \int {e^{-\alpha _ i \left| {{\rm {\bf r}}_{\rm {\bf 1}} -{\rm {\bf A}}} \right|^2}e^{-\beta _ j \left| {{\rm {\bf r}}_{\rm {\bf 1}} -{\rm {\bf B}}} \right|^2}\left[ {\frac{1}{r_{12} }} \right]e^{-\gamma _ k \left| {{\rm {\bf r}}_{\rm {\bf 2}} -{\rm {\bf C}}} \right|^2}e^{-\delta _ l \left| {{\rm {\bf r}}_2 -{\rm {\bf D}}} \right|^2}d{\rm {\bf r}}_{\rm {\bf 1}} d{\rm {\bf r}}_{\rm {\bf 2}} } \nonumber $](images/img-2523.png) |
|||
 |
 |
![$\displaystyle \sum \limits _{i=1}^3 {\sum \limits _{j=1}^3 {\sum \limits _{k=1}^3 {\sum \limits _{l=1}^3 {[s_ i s_ j \vert s_ k s_ l ]} } } } $](images/img-2524.png) |
(B.5) |
and requires 81 primitive integrals for the single ERI. The problem escalates dramatically for more highly contracted sets (STO-6G, 6-311G) and has been the motivation for the development of techniques for shell-pair modeling [930], in which a second shell-pair is constructed with fewer primitives that the first, but introduces no extra error relative to the integral threshold sought.
The Pople-Hehre axis-switch method [922] is excellent for high contraction low angular momentum integral classes.